The bob of a simple pendulum of length $1 \mathrm{m}$ has mass $100 \mathrm{g}$ and a speed of $1.4 \mathrm{m} {\mathrm{s}}^{-1}$ at the lowest point in its path. Find the tension in the string at this instant.

A motorcycle has to move with a constant speed on an overbridge which is in the form of a circular arc of radius $R$ and has a total length $L$. Suppose the motorcycle starts from the highest point.
(a) What can its maximum velocity be for which the contact with the road is not broken at the highest point?
(b) If the motorcycle goes at speed $\frac{1}{\sqrt{2}}$ times the maximum found in part $\left(a\right)$ where will it lose the contact with the road?
(c) What maximum uniform speed can it maintain on the bridge if it does not lose contact anywhere on the bridge?

A block of mass $m$ is kept on a horizontal ruler. The friction coefficient between the ruler and the block is $\mu .$ The ruler is fixed at one end and the block is at a distance $L$ from the fixed end. The ruler is rotated about the fixed end in the horizontal plane through the fixed end.

(a) What can the maximum angular speed be for which the block does not slip? 

(b) If the angular speed of the ruler is uniformly increased from zero at an angular acceleration $\alpha ,$ at what angular speed will the block slip?

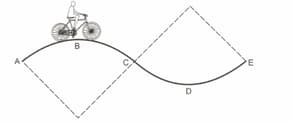
 A track consists of two circular parts $ABC$ and $CDE$ of equal radii $100 \mathrm{m}$, and joined smoothly, as shown in the figure. Each part subtends a right angle at its centre. A cycle weighing $100 \mathrm{kg}$ together with the rider travels at a constant speed of $18 \mathrm{km} {\mathrm{h}}^{-1}$ on the track.

(a) Find the normal contact force by the road on the cycle, when it is at $B$ and at $D$. 

(b) Find the force of friction exerted by the track on the tyres, when the cycle is at $C$. 

(c) Find the normal force between the road and the cycle, just before and just after the cycle crosses $C$.

(d) What should be the minimum friction coefficient between the road and the tyre, which will ensure that the cyclist can move with constant speed? Take $g=10 \mathrm{m} {\mathrm{s}}^{-2}$. 

In a children's park, a heavy rod is pivoted at the center and is made to rotate about the pivot so that the rod always remains horizontal. Two kids hold the rod near the ends and thus rotate with the rod, as shown in the figure. Let the mass of each kid be $15 \mathrm{kg}$, the distance between the points of the rod where the two kids hold it, be $3·0 \mathrm{m}$ and suppose that the rod rotates at the rate of $20$ revolutions per minute. Find the force of friction exerted by the rod on one of the kids.